Space Travel:
Mathematics Uncovers an Interplanetary Superhighway
Lagrange Points
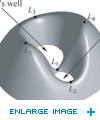In a system consisting of two celestial bodies, say the Earth and the Moon, the combined gravitational wells of the two bodies produce a potential energy surface like the one at left, as seen in a frame of reference turning with the two bodies in their orbit. For over two hundred years, it's been known that there are five points of equilibrium, named Lagrange points after their co-discoverer.
Three points are along the Earth-Moon line: L1 is between them, L2 is on the far side of the Moon, and L3 is on the far side of the Earth. Two other points, L4 and L5, are 60 degrees ahead of and behind the Moon in its orbit. Although each represents a special orbit around the Earth, they are called "points" because they appear as fixed locations when viewed in the reference frame that rotates with the orbit of the two massive bodies. Five special spots exist for every pair of massive bodies in orbit about each other: the Sun and a planet, a planet and one of its moons, and so on. L1 and L2 are of direct interest for understanding the interplanetary transport network, because they form key gateways to faraway destinations.
The motion of a spacecraft near these points is influenced by a delicate interplay of its velocity with the local gravitational field. The richness of the dynamics makes it possible for a spacecraft to "orbit" L1 or L2, even though there is no material object there. Although such orbits around a mere point in space appear very bizarre, they are, in fact, nothing more than near misses to being exactly on L1 or L2 and moving at just the right velocity for perfect balance.
