Space Travel:
Mathematics Uncovers an Interplanetary Superhighway
Mission Design
To have a space mission that can actually fly, you need to find a path through space connecting an initial orbit and a destination orbit. And you need to do this using a reasonable amount of fuel. Think about going from Earth orbit, like the one the International Space Station is in, to an orbit around the Moon.
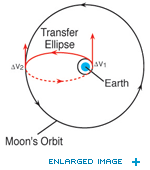You could try getting there in a straightforward way, using an elliptical transfer trajectory from the Earth to the Moon. An upper stage rocket can supply the first large maneuver which burns an amount of fuel proportional to the change in velocity, or ΔV, imparted to the spacecraft. But as it hurtles away from the Earth, the spacecraft still has to have enough on-board fuel to perform a pretty large rocket burn, labeled ΔV2, to get caught into an orbit around the Moon.
A less obvious trajectory to the Moon uses the gravity of the Earth, Moon, and Sun. Heading out about four-times further than the Moon's orbit, the spacecraft feels a curious combination of the Earth and Sun's gravity. The effect is to guide the spacecraft into an orbit around the Moon for free, or "ballistically" in spaceflight lingo. This trajectory uses significantly less on-board fuel than the straightforward approach, but requires more flight time.
Some previous space missions have used this rich interplay of several gravitational fields. By understanding the mathematics governing the paths such missions use, other interesting future missions can be designed, not only in the Earth-Moon system, but around other planets and moons in the solar system.
